How to Calculate Acceleration

When you feel the sudden lurch of a car as it speeds up or the push of your seat while an airplane takes off, you’re experiencing acceleration. In the world of physics, acceleration is a fundamental concept that describes how quickly something is speeding up or slowing down. It’s not just about speed, but about how speed changes over time. Understanding acceleration can be essential for everyone from scientists and engineers to students and everyday individuals. With the right approach, anyone can learn to calculate acceleration and gain a deeper appreciation for the forces at play in our daily lives.

Using Basic Formula

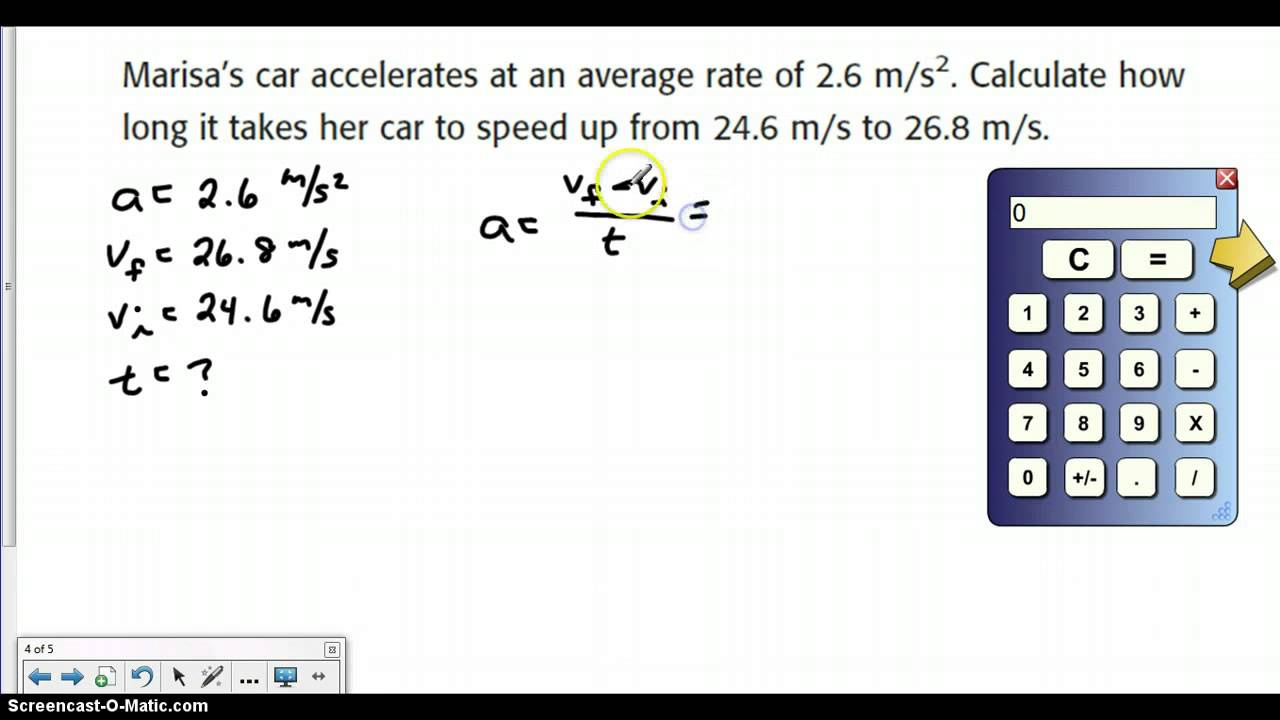
Acceleration is all about figuring out the rate at which an object’s velocity changes. One of the most straightforward ways to calculate it is by using the basic acceleration formula, which is perfect for scenarios with constant acceleration, like a car cruising down the highway at a steady increase in speed.

Detailed Steps:

1. Identify the initial velocity (V), which is the speed at which the object starts.

2. Determine the final velocity (V), which is the speed at which the object ends during the period you’re considering.

3. Calculate the time (t) it takes to change from the initial to the final velocity.

4. Substitue the values into the acceleration formula, which is:

   a = ( frac{(V – V)}{t} )

   Here, ‘a’ represents acceleration.

5. Solve the equation to find the acceleration, typically measured in meters per second squared (m/s).

Summary:
This method is straightforward and works well for simple, linear situations. However, it assumes constant acceleration, which isn’t always the case in real life where acceleration can vary.

Graphical Method

Acceleration can also be calculated using a velocity-time graph, which is an excellent visual tool that helps you understand how velocity changes over time.

Detailed Steps:

1. Draw a velocity-time graph with velocity on the y-axis and time on the x-axis.

2. Plot the points that represent the object’s velocity at various times and connect them with a line to form a curve or a straight line.

3. Find the slope of this line or curve at any point by drawing a tangent.

4. The slope of the tangent line at any given point on the graph will be equal to the acceleration at that time.

5. To find the slope (and thus acceleration), choose two points on the tangent line and use the formula:

   ( text{slope} = frac{text{change in y}}{text{change in x}} )

   The ‘change in y’ corresponds to a change in velocity and ‘change in x’ corresponds to a change in time.

Summary:
This method is more visual and helps to illustrate varying acceleration. However, it demands careful graph plotting and may not be as precise if the graph is not accurately drawn.

Accelerometer Reading

An accelerometer is a tool that measures acceleration directly, typically found in smartphones and various electronic devices. This can be one of the most accurate methods for real-world testing.

Detailed Steps:

1. Understand how your device measures acceleration. It may provide acceleration due to movement, as well as due to gravity.
2. Mount the device securely to the object whose acceleration you wish to measure.
3. Use the device’s app or software to record acceleration data as the object moves.
4. Analyze the data provided by the accelerometer to find the acceleration, being mindful of the direction of movement since acceleration is a vector quantity.

Summary:
While this is a direct and generally accurate method, accelerometers can have limitations in sensitivity and range. They also require the separate analysis of gravitational effects if present.

Using Kinematic Equations

For uniformly accelerated motion, kinematic equations can be used to calculate acceleration when we know other variables related to motion.

Detailed Steps:

1. Review the kinematic equations to find the one that suits the variables you know:

   ( V = V + at ) (where V is final velocity, V is initial velocity, a is acceleration, and t is time)

2. Choose the kinematic equation which allows you to solve for acceleration based on the information you have.

3. Substitute the known values into the equation.

4. Solve for ‘a’ to find the acceleration.

Summary:
Kinematic equations offer a comprehensive approach for various scenarios but require an understanding of the initial conditions of the motion. They also assume constant acceleration.

Inclined Plane

When an object is sliding down an inclined plane, its acceleration can be calculated taking into account gravity and the angle of the incline.

Detailed Steps:

1. Measure the angle (θ) of the incline with respect to the horizontal.
2. Calculate the acceleration due to gravity component along the incline: ( g sin(θ) ), where g is the acceleration due to gravity (9.81 m/s on Earth).
3. For a frictionless incline, this value is the acceleration of the object: ( a = g sin(θ) ).

Summary:
This method simplifies the scenario to an idealistic situation without friction and requires careful measurement of angles.

Pendulum Method

A simple pendulum can provide an indirect method of measuring gravitational acceleration, which is indirectly related to other forms of acceleration.

Detailed Steps:

1. Measure the length (L) of the pendulum.
2. Time how long it takes (T) for the pendulum to complete one full cycle (period).
3. Apply the formula for the period of a pendulum: ( T = 2pi sqrt{frac{L}{g}} ), where ( T ) is the period and ( g ) is the acceleration due to gravity.
4. Rearrange the equation to solve for ( g ), where ( g = frac{4piL}{T} ).

Summary:
While this is a classic physics experiment, it’s indirect and may not accurately calculate other forms of acceleration beyond gravitational.

Using Motion Sensors

Motion sensors, similar to accelerometers, can provide direct measurements of an object’s acceleration.

Detailed Steps:

1. Attach a motion sensor to the object you wish to measure.
2. Use specific software to record the motion data provided by the sensor.
3. Extract the acceleration data from this recording, noting that it will measure all forces acting on the object, adjusting for gravity if necessary.

Summary:
Motion sensors can provide accurate and immediate data, though they can be expensive and require calibration.

Projectile Motion

When an object is thrown into the air and moves under the influence of gravity alone, it’s known as projectile motion.

Detailed Steps:

1. Measure the initial velocity of the object (V) at the angle (θ) it was launched.
2. Determine the time of flight or the time until the object lands.
3. Use kinematic equations to calculate the vertical component of acceleration, which for projectiles on Earth will always be ( g ) (9.81 m/s).

Summary:
Projectile motion is good for illustrating the effects of gravity but is not as applicable in scenarios that don’t involve gravity as a primary force.

Using Force and Mass

You can use Newton’s second law to calculate acceleration if you know the force applied to an object and its mass.

Detailed Steps:

1. Measure the force (F) applied to the object.
2. Determine the mass (m) of the object.
3. Apply Newton’s second law, ( a = F/m ), to find acceleration.

Summary:
This method relates directly to how forces cause acceleration, but it requires accurate measurement of force and mass.

Conservation of Momentum

The conservation of momentum principle can sometimes be used to calculate acceleration, particularly in collisions or explosions.

Detailed Steps:

1. Measure the mass and velocity of objects before and after an event (collision or explosion).
2. Use conservation of momentum equations to determine changes in velocity.
3. From the change in velocity and time over which it occurred, calculate acceleration.

Summary:
This method is more advanced and specific to certain scenarios, and it requires a solid understanding of momentum principles.

In conclusion, acceleration, a fundamental concept in physics, captures how fast an object’s speed changes. From straightforward equations to modern sensor technology, there are numerous ways to calculate it, each with its own benefits and limitations. The key is to understand the context and choose the method that applies best to the situation at hand. Whether you’re a student grappling with academic problems or just curious about the world of motion around you, grasping the concept of acceleration can be incredibly rewarding.

FAQs

Q: Why is it important to know an object’s acceleration?
A: Knowing an object’s acceleration is crucial for understanding and predicting its motion, whether you’re engineering a safe vehicle, tracking the speed of a roller coaster, or simply trying to understand the forces of nature that affect us every day.

Q: Do I always need special equipment to measure acceleration?
A: Not necessarily. While equipment like accelerometers and motion sensors can make the process easier and more accurate, basic calculations can be performed with just a stopwatch and some measurements, depending on the situation.

Q: How does gravity relate to acceleration?
A: Gravity is a force that causes an acceleration of 9.81 m/s towards the Earth’s center. It’s a specific instance of acceleration that affects all objects with mass and is a key factor in many acceleration calculations.