How to Calculate the Square Feet of a Triangle

When it comes to understanding the size and space within shapes, calculating the area is an invaluable skill. With triangles being one of the fundamental shapes you’ll encounter, knowing how to find their square footage is crucial whether you’re planning a garden layout, doing home renovations, or tackling a school project. Unlike measuring a rectangle’s area, which is straightforward multiplication, triangles require a bit more math due to their three-sided nature. However, with a few simple calculations, anyone can become adept at finding the square footage of a triangle.

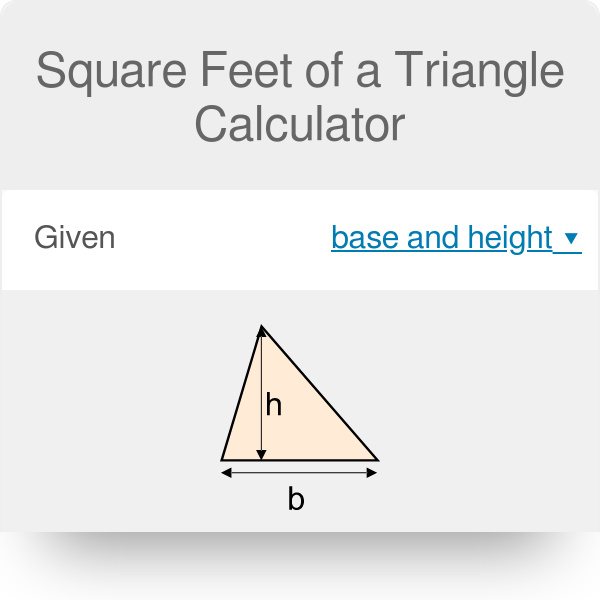
Traditional Method

The traditional way to calculate the area of a triangle involves using its base and height. This method is perhaps the most commonly taught and can be applied to all types of triangles, provided you know these two measurements.

Here’s how to calculate the area using the traditional method:

1. Identify the base of the triangle – this can be any side that you designate as the bottom.
2. Measure the base.
3. Determine the height which is the perpendicular distance from the base to the opposite vertex (the top point of the triangle).
4. Measure the height.
5. Use the formula: Area = 1/2 × base × height.
6. Calculate the area using the measurements obtained.
7. Convert the area into square feet if your measurements weren’t in feet.

This method is excellent for its simplicity and reliability. By only needing to measure two dimensions, it minimizes room for error. However, it can be tough to measure the height of a triangle without right angles or when the triangle is irregular.

Heron’s Formula

Heron’s formula provides a way to calculate the area of a triangle when you know the length of all three sides. This approach is especially useful when the height of the triangle is difficult to measure.

To use Heron’s formula, follow these steps:

1. Measure the length of all three sides of the triangle.
2. Calculate the semi-perimeter (s) of the triangle using the formula: s = (a + b + c) / 2, where a, b, and c are the lengths of the sides.
3. Use Heron’s formula: Area = √(s × (s – a) × (s – b) × (s – c)).
4. Compute the area using the side lengths and the semi-perimeter.

Heron’s formula is versatile since it doesn’t rely on height measurements. It’s particularly useful for irregular shapes, but it requires accurate measurements of all sides and a basic understanding of square roots, which might be a slight complication for some.

Trigonometric Method

When working with right-angled triangles or having knowledge of the angles and sides of the triangle, trigonometry offers another way to calculate the area.

Steps for the trigonometric method include:

1. Identify if the triangle has a right angle. If so, the two sides encasing the right angle are the base and height.
2. If not a right-angled triangle, measure one side to use as the base.
3. Measure the angle adjacent to the base.
4. Measure the other side enclosing the angle.
5. Use the trigonometric formula: Area = 1/2 × base × adjacent side × sin(angle).
6. Calculate the area with the side lengths and angle.

The trigonometric method is valuable when dealing with triangles where the height is not easily measured, or when you’re given angles and side lengths instead. However, it requires knowledge of sine functions and may not be as straightforward for those less familiar with trigonometry.

Using a Grid

For visual learners or hands-on individuals, laying a grid over a scaled drawing of the triangle can assist in approximating the area.

Follow these steps to use a grid:

1. Draw a scaled version of the triangle on graph paper.
2. Count the full squares within the triangle to estimate the area.
3. Approximate the area of the partially filled squares and add them to your count.
4. Sum the total area based on the scale of your graph paper.

This approach is interactive and can be less intimidating for those uncomfortable with mathematical formulas. However, it is less precise and suitable mainly for approximation rather than exact calculations.

Computer Software and Apps

Utilizing technology can simplify the process of calculating the area of a triangle. There are computer programs and mobile apps designed for this purpose.

Here’s how you can use technology:

1. Find and download a reliable app or software that calculates the area of triangles.
2. Input the required dimensions or measurements into the program.
3. Allow the software to compute the area.

This method is quick and reduces the potential for human error. However, it does rely on technology availability, and it might not offer as much learning opportunity compared to manually calculating the area.

Geometric Decomposition

Sometimes breaking a complex shape down into simpler ones can help calculate the area. This is known as geometric decomposition.

To use this technique:

1. Split the triangle into simpler shapes, such as rectangles and right triangles.
2. Calculate the area of these simple shapes individually.
3. Add up the areas of all shapes to get the total area of the original triangle.

This method encourages problem-solving and can clarify the concept of area, but it may not be suitable for all triangle types and could overcomplicate the calculation process for some.

Surveying Tools

For larger triangles, such as a plot of land, using surveying tools can assist in the measurement process.

The steps for using surveying tools include:

1. Use tools like measuring tapes or laser distance meters to measure the sides or the base and height of the triangle.
2. Mark your measurements accurately on a diagram.
3. Calculate the area using these measurements.

This method improves accuracy for large areas but requires access to specialized tools and might not be convenient for smaller, indoor measurements.

Approximation Through Comparison

When precision is not paramount, comparing the triangle to a known area can provide a rough estimate.

To approximate through comparison:

1. Find a shape of known area (like a rectangle) that is similar in size to the triangle.
2. Estimate how many times this known shape fits into the triangle.
3. Derive an approximate area based on this comparison.

This method is quick and requires no complex calculations, making it ideal for estimations. However, it’s not recommended when precise measurements are necessary.

Math Websites and Online Calculators

There are numerous websites designed to assist with mathematical problems that can calculate the area of a triangle easily.

To use these resources:

1. Search for a website that provides an area calculator for triangles.
2. Input your measurements as directed by the site.
3. The website will compute and provide the area.

This method is accessible and user-friendly, offering step-by-step guidance in some cases. However, depending on the site, you may have limited options in terms of the type of triangle measurements you can input.

Scale Drawing and Planimeter

A planimeter is a tool that can measure the area of a shape drawn on paper by tracing the perimeter.

Here’s how you use a planimeter:

1. Draw a scaled-down version of the triangle on paper.
2. Use the planimeter to trace around the perimeter of the triangle.
3. Read the scaled area measurement from the planimeter.

This method can be very precise, yet it does require learning how to operate the planimeter and may not be easily accessible to everyone.

Summary

Calculating the square footage of a triangle can be done through a variety of methods, from traditional and trigonometric formulas to technological solutions and hands-on approaches. Each method has its benefits, like the simplicity of the traditional method or the precision of software calculations, but also potential downsides, such as the complexity of trigonometric calculations or the estimations involved with grid methods.

In conclusion, calculating the square feet of a triangle may seem daunting at first, but once you understand the different methods available, you can confidently tackle this task regardless of the situation. Whether doing it manually with basic measurements or using sophisticated tools, there’s a solution that fits every need and skill level. Just remember to be precise in your measurements and calculations, and you’ll be able to determine the area of a triangle effectively.

Here are some FAQs to help you understand better:

FAQs:

1. What are the most important measurements I need to know to calculate the square footage of a triangle?

• To calculate the square footage of a triangle, you typically need to know the base and the height. However, for some methods, such as Heron’s formula or the trigonometric method, you may need to know the lengths of all three sides or the angles in addition to the sides.

2. Can I use these methods to calculate the area of an irregular triangle?

• Yes, several methods like Heron’s formula, trigonometric calculations, or geometric decomposition can be used to calculate the area of irregular triangles.

3. Do I need special equipment to calculate the square feet of a triangle?

• While most calculations can be completed with simple tools like a ruler and calculator, some methods may require specialized tools like surveying equipment or planimeters for large or more precise measurements.